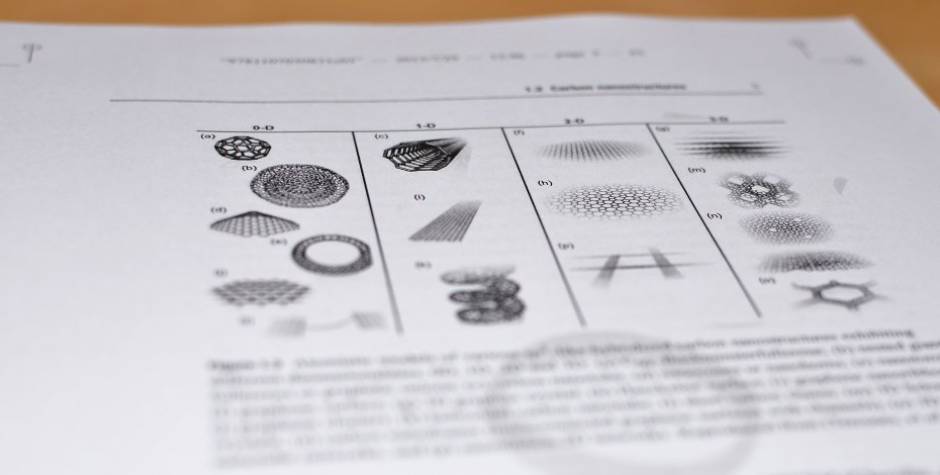
Preview →
introductiontographene.org
421 pages, ca. 50 exercises and problems. Preview it here.
"A very attractive book on graphene-based materials that takes a reader or student with no prior exposure to this topic to a level where he or she can carry out research at a high level and work in this area professionally
I would expect this to become a popular text for present and future researchers."
Mildred Dresselhaus, Massachusetts Institute of Technology
"The book should be useful not only for theoretical researchers but also for graduate students and experimental researchers, who will quickly understand the theorists’ perspective. This book will be an important basic textbook on the physics of graphene."
R. Saito, Tohoku University
"A very attractive book on graphene-based materials that takes a reader or student with no prior exposure to this topic to a level where he or she can carry out research at a high level and work in this area professionally[...] I would expect this to become a popular text for present and future researchers." (read the full review)
Mildred Dresselhaus, Massachusetts Institute of Technology
"The book should be useful not only for theoretical researchers but also for graduate students and experimental researchers, who will quickly understand the theorists’ perspective. This book will be an important basic textbook on the physics of graphene." (read the full review)
R. Saito, Tohoku University
"Overall, this is an exceptional contribution to furthering graphene research, development and applications."
K. Alan Shore, School of Electronic Engineering, Bangor University, Wales
Subscribe now to our newsletter!
Be the first to get updated on the latest news and additions to this website.
Do you have any questions, doubts or comments?
Click here!
Stephan’s recursion methods
One of the most efficient methods available for computing the Density of States (DoS) and the transport properties, specially in samples containing a large number of atoms, is the recursion method explained in Appendix D of “Introduction to Graphene-based Nanomaterials”. Here we provide (by courtesy of Stephan Roche) a numerical code written in fortran and…
Recursive Green’s functions and self-energies
Here we provide a simple fortran 90 code that implements the recursive Green’s functions techniques for calculating the self-energy of a semi-infinite lead as described in Appendix C of “Introduction to Graphene-Based Nanomaterials”. To call this routine you must provide an array with matrices containing the hoppings between the layers as well as the diagonal…
ABINIT input files for graphene nanoribbons
ABINIT input files for armchair and zigzag graphene nanoribbons (by courtesy of Jean-Christophe Charlier). These inputs allow to calculate the structural and electronic properties of these GNRs (including spin-polarization in the zigzag case due to the potential magnetization at the edges). The PAW pseudopotentiels for carbon and hydrogen are also included. agnr.in zgnr.in 1h-gga-uspp.paw 6c-gga-uspp.paw
Python packages for graphene-based materials and beyond
Python is one of the most versatile languages for coding. It allows fast coding of complex algorithms and the community working with Python is growing at a fast pace. Here we recommend two packages written for python: PythTB: Python Tight-Binding is a package developed at Rutgers University by the group of David Vanderbilt. It allows to compute…